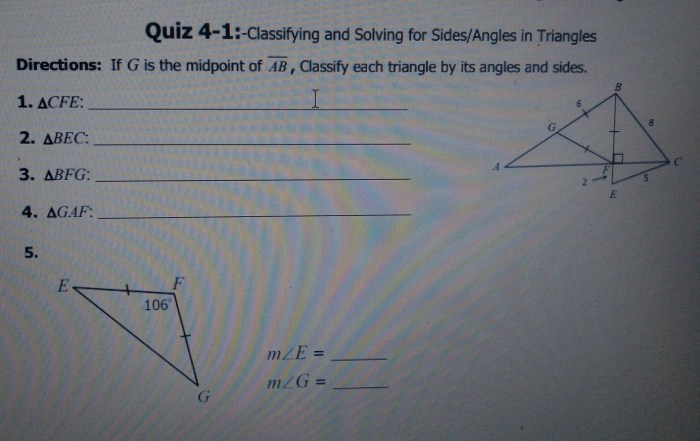
Quiz 4-1 classifying and solving for sides/angles in triangles – Introducing quiz 4-1: classifying and solving for sides and angles in triangles. This engaging exploration delves into the fundamental concepts of triangles, empowering you with the knowledge to conquer triangle-related challenges with precision and confidence.
Delve into the realm of triangles, where we unravel their defining characteristics and delve into the intricacies of classifying them based on their sides and angles. Prepare to master the art of solving for unknown sides and angles, unlocking the secrets that lie within these geometric wonders.
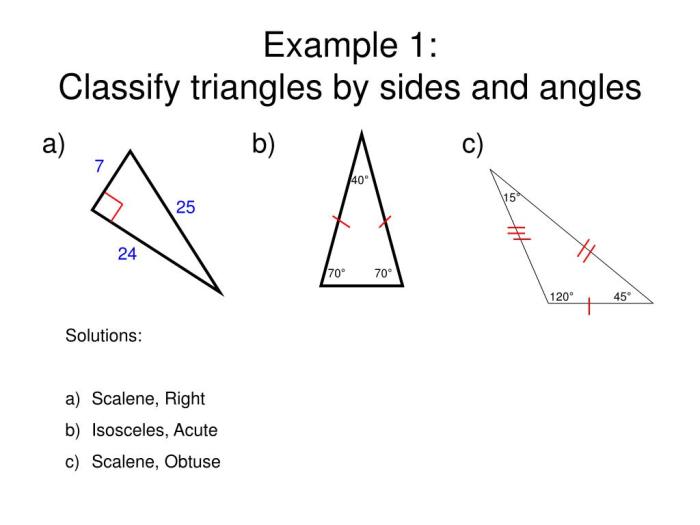
Classifying and Solving for Sides/Angles in Triangles
Triangles are fundamental geometric shapes consisting of three sides and three angles. Understanding their properties and relationships is crucial in various fields, including engineering, architecture, and navigation.
Triangles can be classified based on the length of their sides and the measure of their angles. This classification helps in identifying specific properties and relationships among the sides and angles.
Classifying Triangles, Quiz 4-1 classifying and solving for sides/angles in triangles
| Type | Sides | Angles |
|---|---|---|
| Equilateral | All three sides equal | All three angles equal (60°) |
| Isosceles | Two sides equal | Two angles equal |
| Scalene | No sides equal | No angles equal |
| Right | One angle is 90° | Sum of other two angles is 90° |
| Obtuse | One angle is greater than 90° | Sum of other two angles is less than 90° |
| Acute | All angles are less than 90° | Sum of all three angles is 180° |
Question Bank: Quiz 4-1 Classifying And Solving For Sides/angles In Triangles
What are the different types of triangles?
Triangles are classified based on their sides (scalene, isosceles, equilateral) and angles (acute, right, obtuse).
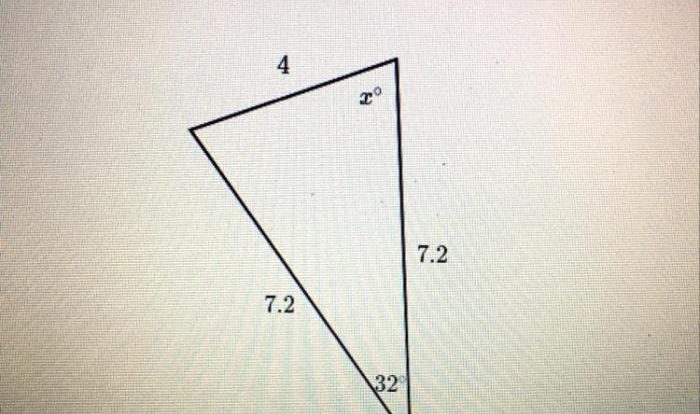
How do I solve for an unknown side in a triangle?
Use the Pythagorean theorem (a^2 + b^2 = c^2) or trigonometric ratios (sine, cosine, tangent).
What are the practical applications of triangle classification and solving?
Engineering design, architecture, navigation, surveying, and more.